Periodic Tiling Patterns
Periodic Tiling Patterns - Web such periodic tilings may be classified by the number of orbits of vertices, edges and tiles. Web covering a flat surface (the plane) with some pattern of geometric shapes (tiles), with no overlaps or gaps, is called a tiling. He is an editor of the journal of mathematics and the arts (formerly chief editor), and an. Web penrose tilings play a central role in the theory because they can be generated by any of the three main methods: Web there are two types of tiling patterns, periodic and aperiodic. Web mathematicians want to know when it’s possible to form aperiodic tiling patterns — patterns like the penrose tilings, which never repeat. Such a shape is called an einstein, a word play on ein stein, german for one stone. Web the tilings obtained from an aperiodic set of tiles are often called aperiodic tilings, though strictly speaking it is the tiles themselves that are aperiodic. A periodic tiling pattern is defined as setting tiles in the same manner at regular intervals. Only three regular polygons tile the plane; Web there are two types of tiling patterns, periodic and aperiodic. Equilateral triangles, squares and hexagons. Several variants of the problem, depending on the particular definitions of non… Web copies of these two tiles can form infinitely many different patterns that go on forever, called penrose tilings. Local matching rules (\jigsaw puzzle); Yet no matter how you arrange the tiles, you’ll never get a. Web the new façade of storey hall in melbourne, by the architects arm, is covered in a particular set of giant aperiodic tessellations which were discovered by the. Equilateral triangles, squares and hexagons. These patterns often symbolize harmony, unity, and the. Web the tilings obtained from an aperiodic. A periodic tiling is one on which you can outline a region of the tiling and tile the plane by translating copies of that region (without rotating or reflecting). Such a shape is called an einstein, a word play on ein stein, german for one stone. Web copies of these two tiles can form infinitely many different patterns that go. Yet no matter how you arrange the tiles, you’ll never get a. Tessellations formed by aperiodic tiles are called aperiodic tessellations. A tessellation is periodic if it has translational symmetry, i.e. He is an editor of the journal of mathematics and the arts (formerly chief editor), and an. Such a shape is called an einstein, a word play on ein. Yet no matter how you arrange the tiles, you’ll never get a. In plane geometry, the einstein problem asks about the existence of a single prototile that by itself forms an aperiodic set of prototiles; A periodic tessellation is a highly ordered pattern. Web copies of these two tiles can form infinitely many different patterns that go on forever, called. Azulejos tiles often include geometric designs like arabesques, stars, and interlocking shapes. In plane geometry, the einstein problem asks about the existence of a single prototile that by itself forms an aperiodic set of prototiles; Only three regular polygons tile the plane; Web the tilings obtained from an aperiodic set of tiles are often called aperiodic tilings, though strictly speaking. Web after 60 years of searching, mathematicians might have finally found a true single ‘aperiodic’ tile — a shape that can cover an infinite plane, but never make a. Web copies of these two tiles can form infinitely many different patterns that go on forever, called penrose tilings. Several variants of the problem, depending on the particular definitions of non…. Web it's the perfect illustration of what mathematicians call a periodic tiling of space, with shapes covering an entire area with no overlap or gap. Equilateral triangles, squares and hexagons. Yet no matter how you arrange the tiles, you’ll never get a. Web the tilings obtained from an aperiodic set of tiles are often called aperiodic tilings, though strictly speaking. Only three regular polygons tile the plane; (the tiling itself is said to be. Tessellations formed by aperiodic tiles are called aperiodic tessellations. In plane geometry, the einstein problem asks about the existence of a single prototile that by itself forms an aperiodic set of prototiles; Web mathematicians want to know when it’s possible to form aperiodic tiling patterns —. As can be seen triangles and squares have strips. (the tiling itself is said to be. Web there are two types of tiling patterns, periodic and aperiodic. Web copies of these two tiles can form infinitely many different patterns that go on forever, called penrose tilings. The most familiar tilings, such as covering a floor with. A periodic tessellation is a highly ordered pattern. These patterns often symbolize harmony, unity, and the. Web copies of these two tiles can form infinitely many different patterns that go on forever, called penrose tilings. Web the tilings obtained from an aperiodic set of tiles are often called aperiodic tilings, though strictly speaking it is the tiles themselves that are aperiodic. Equilateral triangles, squares and hexagons. Only three regular polygons tile the plane; Tessellations formed by aperiodic tiles are called aperiodic tessellations. (the tiling itself is said to be. Several variants of the problem, depending on the particular definitions of non… Web mathematicians want to know when it’s possible to form aperiodic tiling patterns — patterns like the penrose tilings, which never repeat. A tessellation is periodic if it has translational symmetry, i.e. Local matching rules (\jigsaw puzzle); In plane geometry, the einstein problem asks about the existence of a single prototile that by itself forms an aperiodic set of prototiles; Yet no matter how you arrange the tiles, you’ll never. The most familiar tilings, such as covering a floor with. Web covering a flat surface (the plane) with some pattern of geometric shapes (tiles), with no overlaps or gaps, is called a tiling.
Octagon from rhomb, square, octagon tiling 4 Pattern blocks, Octagon

Downloadable Periodic Table Circle Tiles

Tegula exploring a galaxy of twodimensional periodic tilings DeepAI

Aperiodic Tiling order, rhythm and pattern
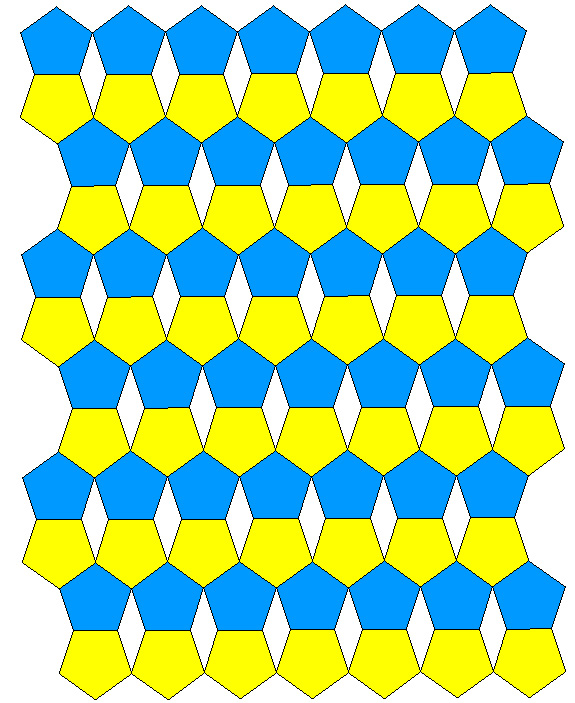
Tiling the plane, periodic and aperiodic
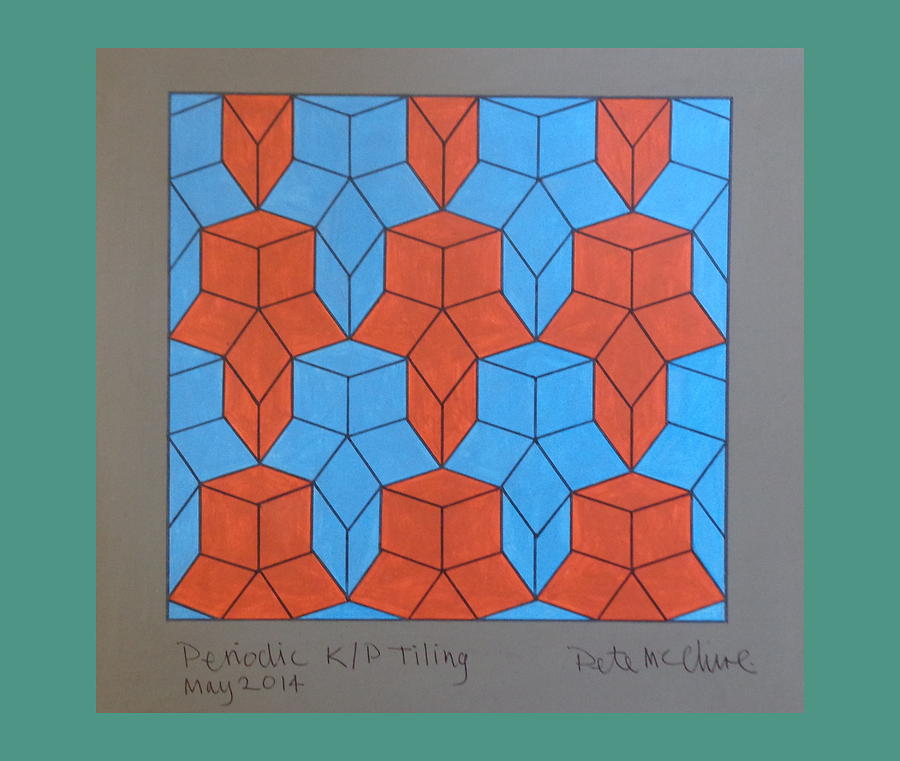
Periodic Tiling Pattern.2014 Painting by Peter Mcclure

FileAcadem Regular hexagons and equilateral triangles in periodic

Patterns of the regular pentagon tiling. (a), (b) Two patterns of
PPT Patterns in the Periodic Table PowerPoint Presentation, free

Downloadable Periodic Table Circle Tiles
Such A Shape Is Called An Einstein, A Word Play On Ein Stein, German For One Stone.
Web Copies Of These Two Tiles Can Form Infinitely Many Different Patterns That Go On Forever, Called Penrose Tilings.
Yet No Matter How You Arrange The Tiles, You’ll Never Get A.
That Is, A Shape That Can Tessellate Space But Only In A Nonperiodic Way.
Related Post: