Patterns In Pascals Triangle
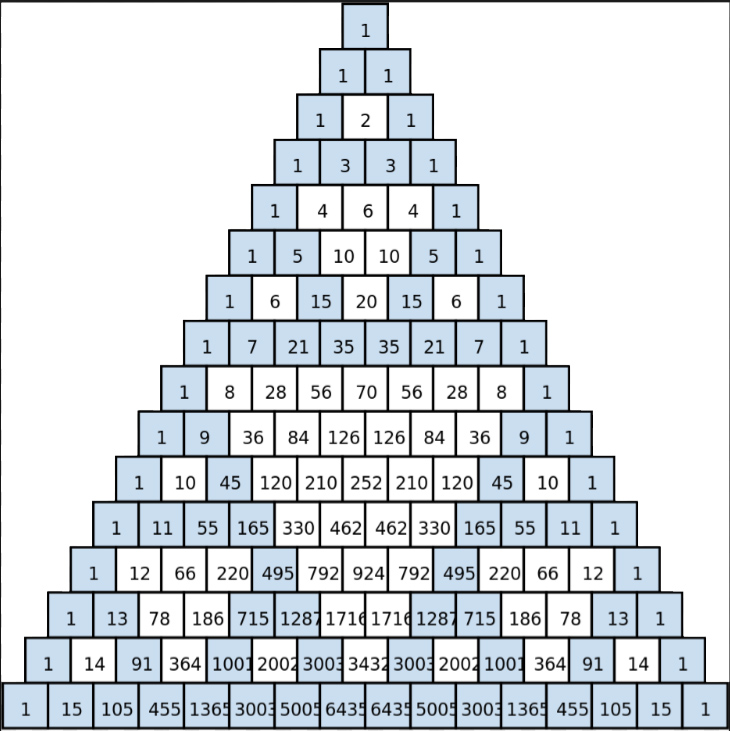
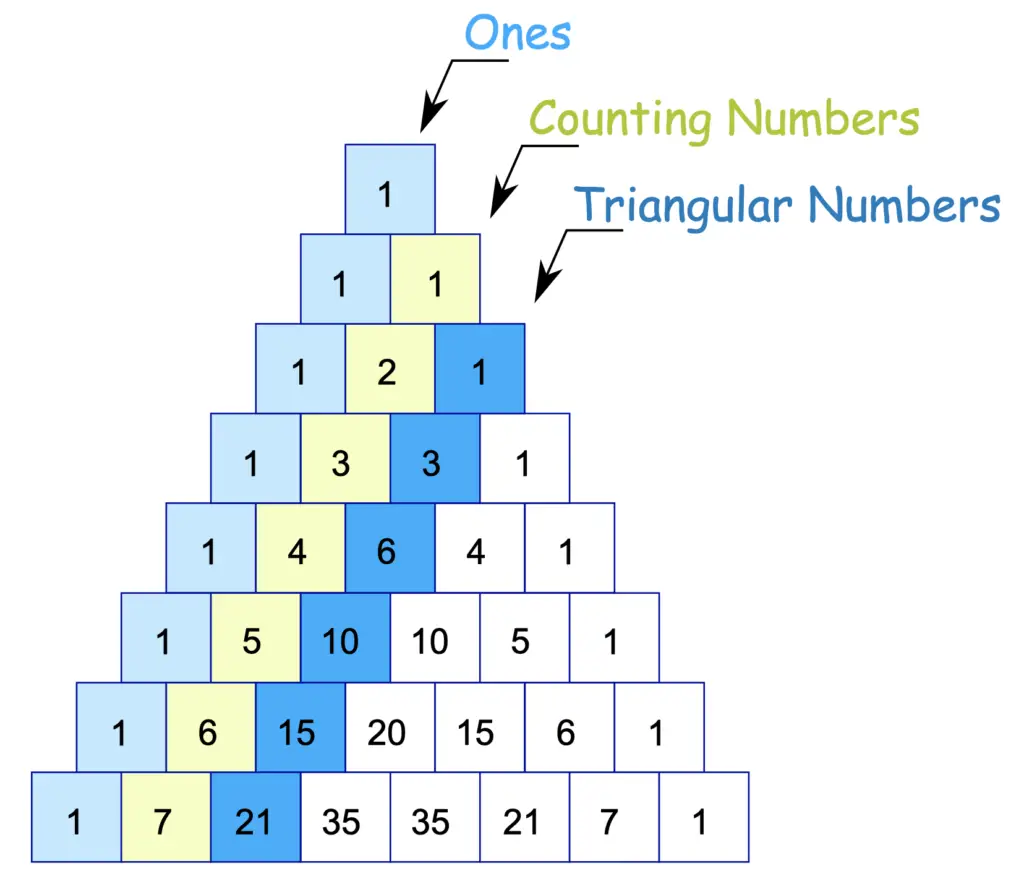
Patterns In Pascals Triangle - Each frame represents a row in pascal's triangle. The first diagonal is ‘1’. Web learn about pascal's triangle, including what it is and how to use it to find coefficients in a binomial expansion in algebra. Expand \((x+y)^4\) using pascal's triangle. For example, in the 4th row 1 4 6 4 1, sum of the elements is 1 + 4 + 6 + 4 + 1 = 16 = 2 4. In terms of the binomial coefficients, cnm =cnn−m. The \(n^\text{th}\) row of pascal's triangle contains the coefficients of the expanded polynomial \((x+y)^n\). Web pascal's triangle is a way to visualize many patterns involving the binomial coefficient. Each column of pixels is a number in binary with the least significant bit at the bottom. Web learn about some of the most fascinating patterns in mathematics, from triangle numbers to the fibonacci sequence and pascal’s triangle. It has a number of different uses throughout mathematics and statistics, but in the context of polynomials, specifically binomials, it is used for expanding binomials. The sum of values in the n th row is 2 n. This interesting pattern and property is named after blaise pascal and has been a famous triangle in mathematics due to its extensive application. In terms of the binomial coefficients, cnm =cnn−m. This follows from the formula for the binomial coefficient. Web learn about some of the most fascinating patterns in mathematics, from triangle numbers to the fibonacci sequence and pascal’s triangle. Expand \((x+y)^4\) using pascal's triangle. Web pascal's triangle is an array of numbers that represents a number pattern. This follows from the formula for the binomial coefficient. It has a number of different uses throughout mathematics and statistics, but in the context of polynomials, specifically binomials, it is used for expanding binomials. To build the triangle, start with 1 at the top, then continue placing numbers below it in a triangular pattern. In terms of the binomial coefficients,. The sum of values in the n th row is 2 n. A few of the pascal triangle patterns are: Each frame represents a row in pascal's triangle. The first diagonal is ‘1’. Web pascal's triangle is an array of numbers that represents a number pattern. In terms of the binomial coefficients, cnm =cnn−m. The sum of values in the n th row is 2 n. To build the triangle, start with 1 at the top, then continue placing numbers below it in a triangular pattern. Web pascal's triangle has various patterns within the triangle which were found and explained by pascal himself or were known. To build the triangle, start with 1 at the top, then continue placing numbers below it in a triangular pattern. In terms of the binomial coefficients, cnm =cnn−m. Web learn about pascal's triangle, including what it is and how to use it to find coefficients in a binomial expansion in algebra. A few of the pascal triangle patterns are: The. It has a number of different uses throughout mathematics and statistics, but in the context of polynomials, specifically binomials, it is used for expanding binomials. Web pascal's triangle has various patterns within the triangle which were found and explained by pascal himself or were known way before him. Web learn about pascal's triangle, including what it is and how to. Expand \((x+y)^4\) using pascal's triangle. It has a number of different uses throughout mathematics and statistics, but in the context of polynomials, specifically binomials, it is used for expanding binomials. This follows from the formula for the binomial coefficient. For example, in the 4th row 1 4 6 4 1, sum of the elements is 1 + 4 + 6. Each column of pixels is a number in binary with the least significant bit at the bottom. Web pascal’s triangle has different patterns within the triangle. The first diagonal is ‘1’. Expand \((x+y)^4\) using pascal's triangle. Web pascal's triangle is an array of numbers that represents a number pattern. Expand \((x+y)^4\) using pascal's triangle. Some of them are listed: Web a really interesting number patterns is pascal's triangle (named after blaise pascal, a famous french mathematician and philosopher). Web pascal's triangle conceals a huge number of various patterns, many discovered by pascal himself and even known before his time. Web pascal's triangle is an array of numbers that represents. For example, in the 4th row 1 4 6 4 1, sum of the elements is 1 + 4 + 6 + 4 + 1 = 16 = 2 4. Some of them are listed: This follows from the formula for the binomial coefficient. Web learn about some of the most fascinating patterns in mathematics, from triangle numbers to the fibonacci sequence and pascal’s triangle. The first diagonal is ‘1’. Each column of pixels is a number in binary with the least significant bit at the bottom. This interesting pattern and property is named after blaise pascal and has been a famous triangle in mathematics due to its extensive application in. Expand \((x+y)^4\) using pascal's triangle. Each frame represents a row in pascal's triangle. In terms of the binomial coefficients, cnm =cnn−m. It has a number of different uses throughout mathematics and statistics, but in the context of polynomials, specifically binomials, it is used for expanding binomials. The \(n^\text{th}\) row of pascal's triangle contains the coefficients of the expanded polynomial \((x+y)^n\). Web pascal's triangle is an array of numbers that represents a number pattern. A few of the pascal triangle patterns are: Web pascal's triangle is a way to visualize many patterns involving the binomial coefficient. Web pascal's triangle conceals a huge number of various patterns, many discovered by pascal himself and even known before his time.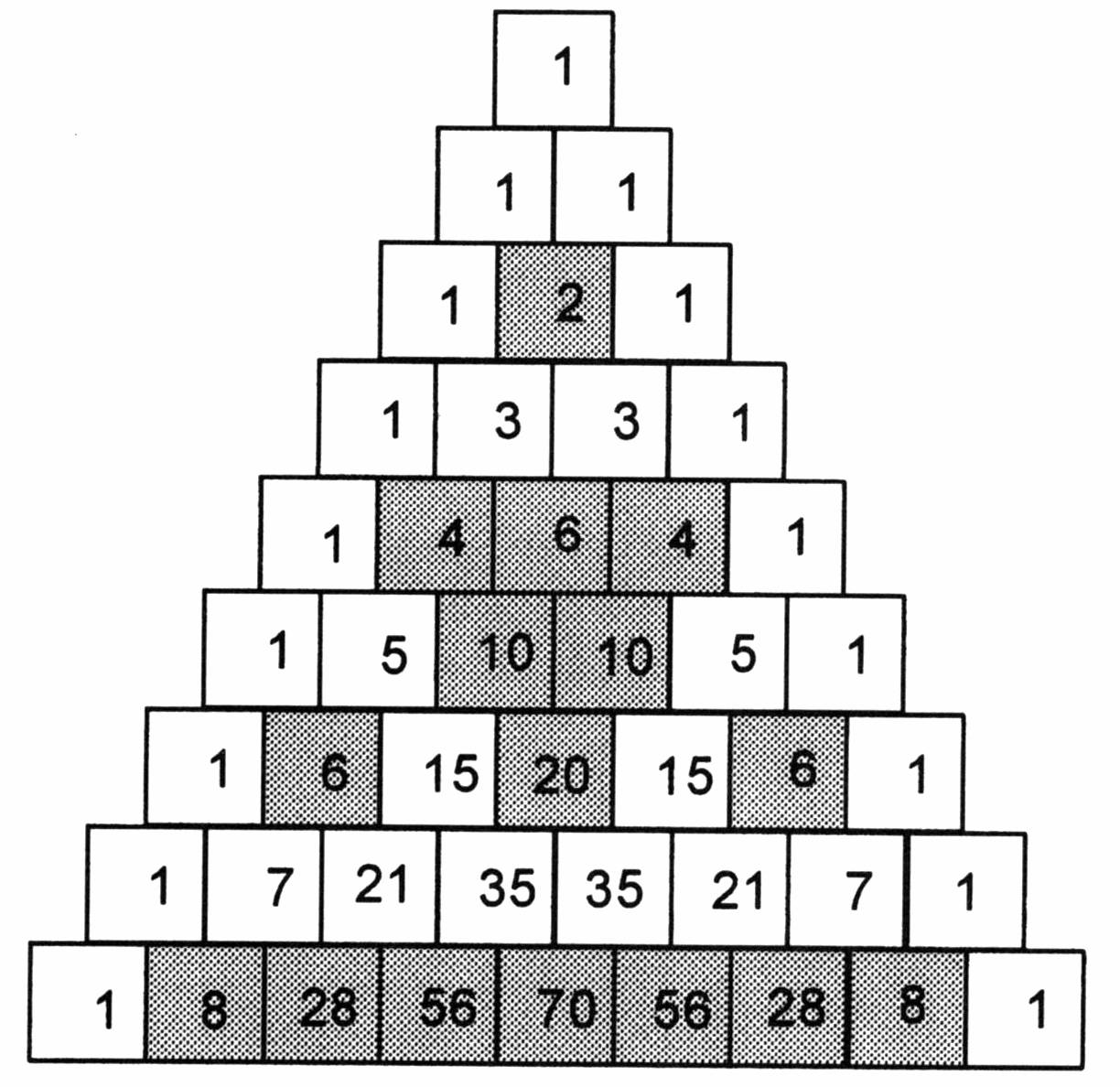
The Mathematical Tourist Pascal's Patterns

Pascal's Triangle Definition, History, Patterns and its Correlations
Pascals Triangle Pascals Triangle Pattern With Flowchart And Images
How to implement the Pascal Triangle in Python practice with loops and

Science Visualized • NUMBER PATTERNS PASCAL’S TRIANGLE Pascal’s...
Pascal's Triangle Definition, Formula, Patterns, and Examples

Pascal's Triangle Definition, History, Patterns and its Correlations

Pascals Triangle Pascals Triangle Pattern With Flowchart And Images

Pascal's Triangle (68) YouCubed

Interesting Facts About Pascal's Triangle Owlcation
The Sum Of Values In The N Th Row Is 2 N.
Web Pascal's Triangle Has Various Patterns Within The Triangle Which Were Found And Explained By Pascal Himself Or Were Known Way Before Him.
Web Pascal’s Triangle Has Different Patterns Within The Triangle.
Here Are Some Of The Ways This Can Be Done:
Related Post:
