How To Draw A Hyperbola
How To Draw A Hyperbola - Web learn how to graph hyperbolas. Solve for the coordinates of the foci using the equation c =±√a2 +b2 c = ± a 2 + b 2. The central rectangle and asymptotes provide the framework needed to sketch an accurate graph of the hyperbola. Creating a rectangle to graph a hyperbola with asymptotes. A hyperbola is the set of all points (x, y) (x, y) in a plane such that the difference of the distances between (x, y) (x, y) and the foci is a positive constant. If the coefficient of \(x^{2}\) is positive, draw the branches of the hyperbola opening left and right through the points determined by \(a\). Web use these points to draw the fundamental rectangle; The line through the foci, is called the transverse axis. The lines through the corners of this rectangle are the asymptotes. Sticking with the example hyperbola. Web use these points to draw the fundamental rectangle; The lines through the corners of this rectangle are the asymptotes. Beginning at each vertex separately, draw the curves that approach the asymptotes the farther away from the vertices the curve gets. Web the equations of the asymptotes are y = ±a b(x−h)+k y = ± a b ( x −. Use the hyperbola formulas to find the length of the major axis and minor axis. To determine the foci you can use the formula: Solve for the coordinates of the foci using the equation c =±√a2 +b2 c = ± a 2 + b 2. Label the foci and asymptotes, and draw a smooth curve to form the hyperbola, as. Web the equations of the asymptotes are y = ±a b(x−h)+k y = ± a b ( x − h) + k. The line through the foci, is called the transverse axis. The two lines that the. The graph approaches the asymptotes but never actually touches them. Web use these points to draw the fundamental rectangle; Sticking with the example hyperbola. This is the axis on which the two foci are. Solve for the coordinates of the foci using the equation c =±√a2 +b2 c = ± a 2 + b 2. Web this step gives you two lines that will be your asymptotes. A 2 + b 2 = c 2. Solve for the coordinates of the foci using the equation c =±√a2 +b2 c = ± a 2 + b 2. Beginning at each vertex separately, draw the curves that approach the asymptotes the farther away from the vertices the curve gets. Using the hyperbola formula for the length of the major and minor axis. Creating a rectangle to graph. Remember to switch the signs of the numbers inside the parentheses, and also remember that h is inside the parentheses with x, and v is inside the parentheses with y. Web like the ellipse, the hyperbola can also be defined as a set of points in the coordinate plane. Use the hyperbola formulas to find the length of the major. Label the foci and asymptotes, and draw a smooth curve to form the hyperbola, as shown in figure 8. Use the hyperbola formulas to find the length of the major axis and minor axis. Sticking with the example hyperbola. The two points where the transverse axis intersects the hyperbola are each a vertex of. Web use these points to draw. A 2 + b 2 = c 2. The line through the foci, is called the transverse axis. Label the foci and asymptotes, and draw a smooth curve to form the hyperbola, as shown in figure 8. A hyperbola is all points in a plane where the difference of their distances from two fixed points is constant. Beginning at each. Web the equations of the asymptotes are y = ±a b(x−h)+k y = ± a b ( x − h) + k. Using the hyperbola formula for the length of the major and minor axis. To graph a hyperbola from the equation, we first express the equation in the standard form, that is in the form: Web to graph a. Web use these points to draw the fundamental rectangle; Creating a rectangle to graph a hyperbola with asymptotes. Use the hyperbola formulas to find the length of the major axis and minor axis. The central rectangle and asymptotes provide the framework needed to sketch an accurate graph of the hyperbola. Beginning at each vertex separately, draw the curves that approach. Web use these points to draw the fundamental rectangle; Web the equations of the asymptotes are y = ±a b(x−h)+k y = ± a b ( x − h) + k. If the coefficient of \(x^{2}\) is positive, draw the branches of the hyperbola opening left and right through the points determined by \(a\). Label the foci and asymptotes, and draw a smooth curve to form the hyperbola, as shown in figure 8. Using the hyperbola formula for the length of the major and minor axis. Remember to switch the signs of the numbers inside the parentheses, and also remember that h is inside the parentheses with x, and v is inside the parentheses with y. The line through the foci, is called the transverse axis. The two points where the transverse axis intersects the hyperbola are each a vertex of. This is the axis on which the two foci are. Web this step gives you two lines that will be your asymptotes. To graph a hyperbola from the equation, we first express the equation in the standard form, that is in the form: The central rectangle and asymptotes provide the framework needed to sketch an accurate graph of the hyperbola. Length of major axis = 2a, and length of minor axis = 2b. Each of the fixed points is called a focus of the hyperbola. Sticking with the example hyperbola. A hyperbola is the set of all points (x, y) (x, y) in a plane such that the difference of the distances between (x, y) (x, y) and the foci is a positive constant.
How to Draw a HYPERBOLA in Engineering Drawing What is a Hyperbola

Easy Steps to Draw A Hyperbola using Focus Directrix Method Engg
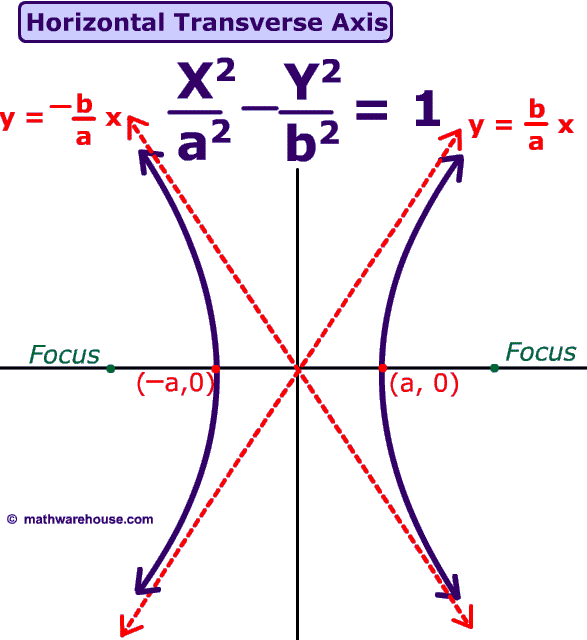
Formula and graph of a hyperbola. How to graph a hyperbola based on its

HOW TO DRAW THE RECTANGULAR HYPERBOLA IN ENGINEERING DRAWING YouTube

HOW TO DRAW A HYPERBOLA USING LOCUS METHOD LOCI ENGINEERING
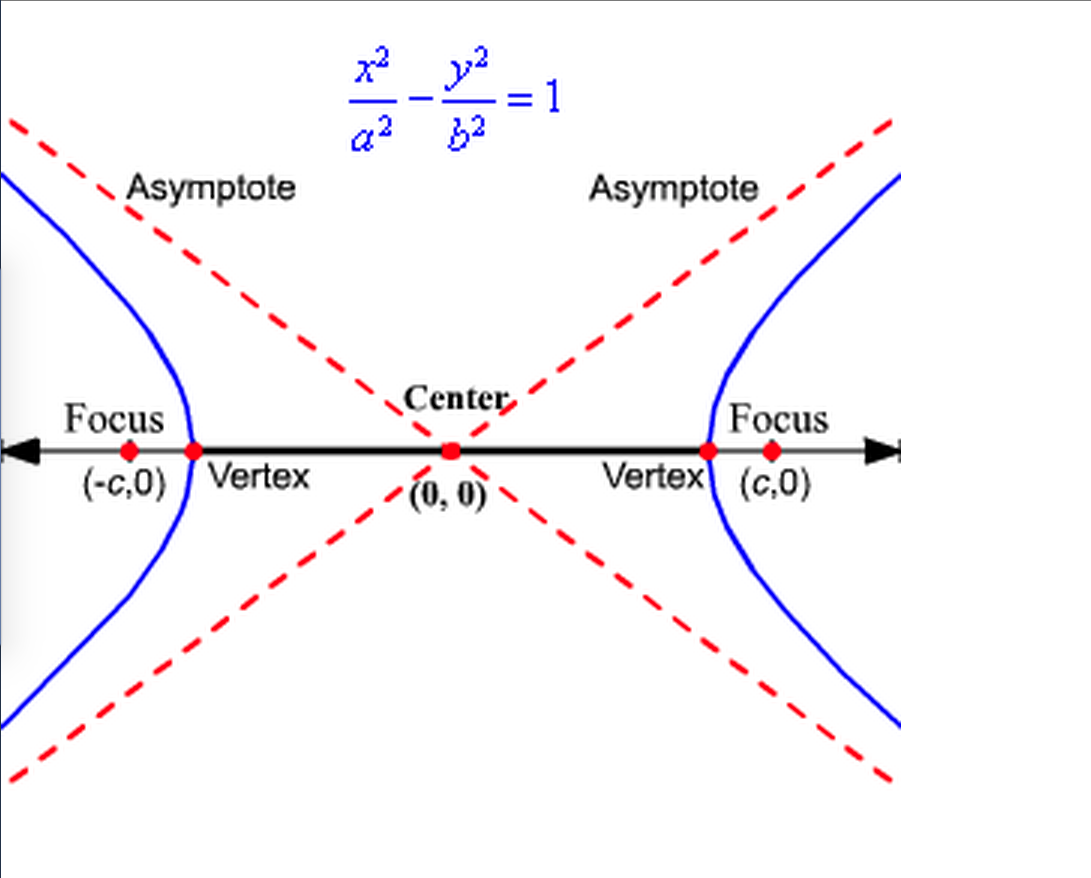
What is a hyperbola?

How to draw a Hyperbola by Arcs of Circle Method YouTube
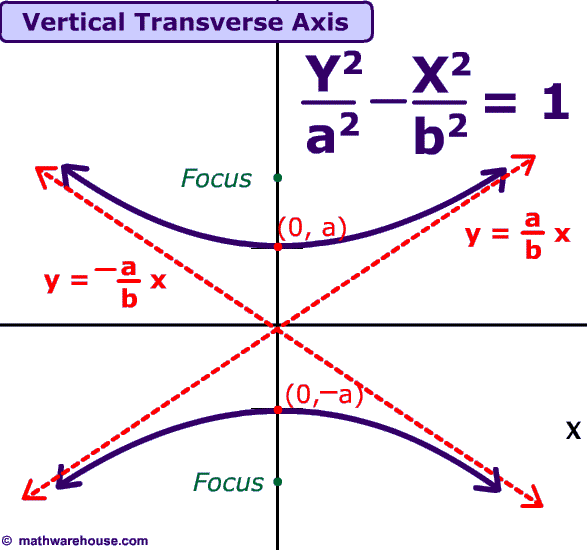
Formula and graph of a hyperbola. How to graph a hyperbola based on its

Easy Steps to Draw A Hyperbola using Focus Directrix Method Engg
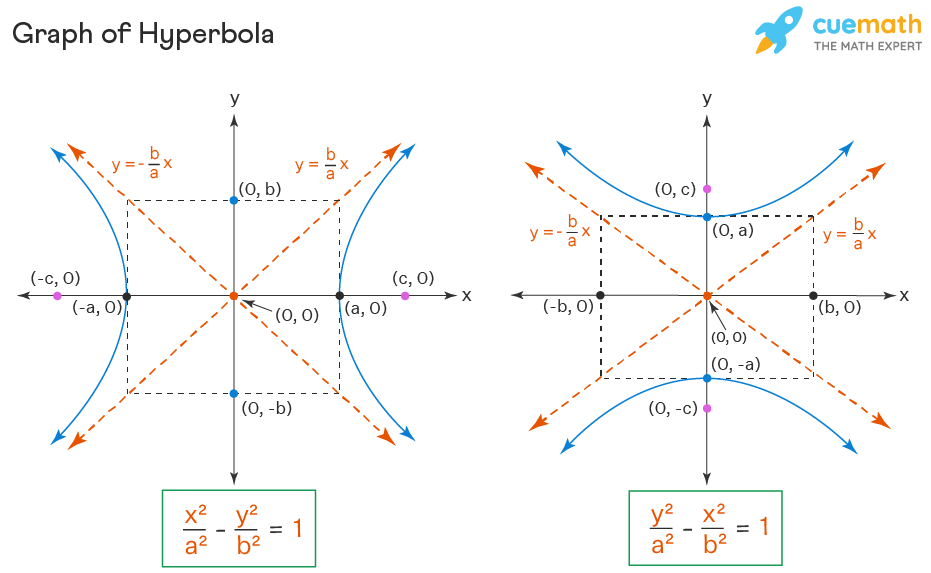
Hyperbola Equation, Properties, Examples Hyperbola Formula
Notice That The Definition Of A Hyperbola Is Very Similar To That Of An Ellipse.
A 2 + B 2 = C 2.
Web Sketch And Extend The Diagonals Of The Central Rectangle To Show The Asymptotes.
Solve For The Coordinates Of The Foci Using The Equation C =±√A2 +B2 C = ± A 2 + B 2.
Related Post: