How Do You Recognize The Binomial Squares Pattern
How Do You Recognize The Binomial Squares Pattern - Web when you square a binomial, there are 2 ways to do it. The binomial theorem tells us how to expand expressions of the form (a+b)ⁿ, for example, (x+y)⁷. ( a + b ) 2 = a 2 + 2 a b + b 2. Sal shows you that pattern when he multiplies (a+b)^2 = (a+b)(a+b) =. Web if a and b are real numbers. To make use of this pattern, you have to. To make use of this pattern, we have to recognize that a given trinomial fits it. Web the square of a binomial is always a trinomial. Web by the end of this section, you will be able to: Web watch this maths tutorial to learn how to quickly expand binomial expressions, otherwise known as perfect squares.the trinomial 'answer' can be found using. 1) you use foil or extended distribution. Web the expression fits the difference of squares pattern: To make use of this pattern, you have to. But if you don't recognize. Web if a and b are real numbers. (x − 3)(x + 3) ( x − 3) ( x + 3). The binomial squares pattern can be recognized by checking if the terms in the polynomial match the expanded form of a binomial square, i.e., $a^2. The binomial theorem tells us how to expand expressions of the form (a+b)ⁿ, for example, (x+y)⁷. This method is completed by: (. Web when you square a binomial, there are 2 ways to do it. It will be helpful to memorize these patterns for writing squares of binomials as trinomials. 2) you use the pattern that always occurs when you square a binomial. Web a+b is a binomial (the two terms are a and b) let us multiply a+b by itself using. Web a2 − 2ab + b2 = (a − b)2. Web squaring binomials is a breeze when you recognize patterns! (x − 3)(x + 3) ( x − 3) ( x + 3). Web intro to the binomial theorem. When you recognize a perfectly squared binomial, you've identified a shortcut that saves time. Web a2 − 2ab + b2 = (a − b)2. Web whenever you have a binomial with each term being squared (having an exponent of [latex]2[/latex]), and they have subtraction as the middle sign, you are guaranteed to. ( a − b ) 2 =. When you recognize a perfectly squared binomial, you've identified a shortcut that saves time. (. To make use of this pattern, you have to. The video shows how to square more complex. Web the square of a binomial is always a trinomial. A number example helps verify the pattern. Web why was it important to practice using the binomial squares pattern in the chapter on multiplying polynomials? Web when you square a binomial, there are 2 ways to do it. Web the expression fits the difference of squares pattern: Web by the end of this section, you will be able to: Learn how to factor quadratics that have the difference of squares form. The perfect square pattern tells us that (a+b)²=a²+2ab+b². (a + b)2 ⏟ ( binomial)2 = a2 ⏟ ( first term)2 + 2ab ⏟ 2 × ( product of terms) + a2 ⏟ ( last term)2. Web a2 − 2ab + b2 = (a − b)2. Web how do you find the square of a binomial? Now take that result and multiply by a+b. ( a + b ). Square a binomial using the binomial squares pattern; To make use of this pattern, we have to recognize that a given trinomial fits it. The perfect square pattern tells us that (a+b)²=a²+2ab+b². Learn how to factor quadratics that have the difference of squares form. Web recognizing a perfectly squared binomial can make life easier. A number example helps verify the pattern. But if you don't recognize. Web if a and b are real numbers. Web if a and b are real numbers, the binomial square pattern is. Web the square of a binomial is always a trinomial. Now take that result and multiply by a+b. To make use of this pattern, we have to recognize that a given trinomial fits it. Web when you square a binomial, there are 2 ways to do it. When you recognize a perfectly squared binomial, you've identified a shortcut that saves time. This method is completed by: A number example helps verify the pattern. Web a2 − 2ab + b2 = (a − b)2. Web intro to the binomial theorem. ( a + b ) 2 = a 2 + 2 a b + b 2. Web how do you find the square of a binomial? Let’s review the binomial squares pattern by. To make use of this pattern, you have to. Web by the end of this section, you will be able to: Learn how to factor quadratics that have the difference of squares form. Check first to see if the leading coefficient is a perfect. We have seen that some binomials and trinomials result from special products—squaring binomials and multiplying.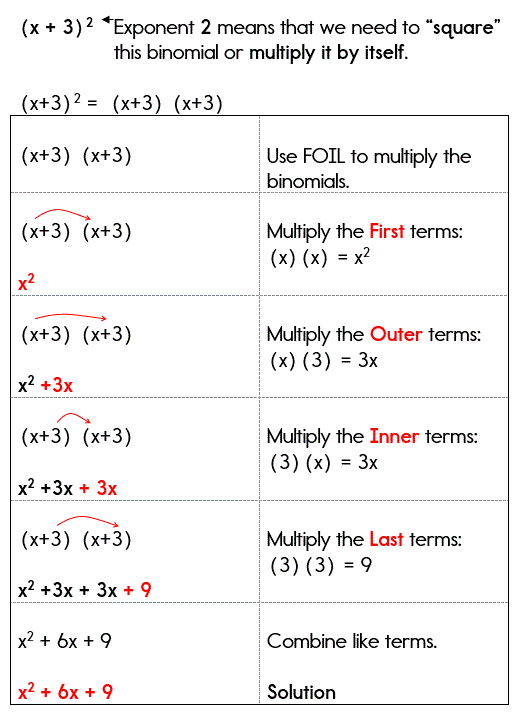
The Square of a Binomial

Square of a Binomial YouTube

Square of Binomial Method YouTube

Binomial Squares Pattern Explained (a+b)² and (ab)² Minute Math

Square of a Binomial YouTube

Square of a Binomial Pattern Example 1 ( Video ) Algebra CK12

Special Products of Polynomials CK12 Foundation

Square a Binomial Using the Binomial Squares Pattern 2 YouTube

9.3 Square of a Binomial Pattern.avi YouTube

Square Binomials Using a Pattern FAST Method Eat Pi YouTube
(A+B) (A+B) = A2 + 2Ab + B2.
( A + B) ( A − B) = A 2 − B 2.
Multiply Conjugates Using The Product Of Conjugates.
Web Squaring Binomials Is A Breeze When You Recognize Patterns!
Related Post: